Position Vectors
Position Vectors Revision
Position Vectors
A position vector describes where a point lies, in relation to the origin, \boldsymbol{O}
This allows you to write other vectors in terms of position vectors.
Make sure you are happy with the following topics before continuing.
A Level Maths Predicted Papers 2024
The MME A level maths predicted papers are an excellent way to practise, using authentic exam style questions that are unique to our papers. Our examiners have studied A level maths past papers to develop predicted A level maths exam questions in an authentic exam format. The profit from every pack is reinvested into making free content on MME, which benefits millions of learners across the country.
A Level Maths Revision Cards
The best A level maths revision cards for AQA, Edexcel, OCR, MEI and WJEC. MME is here to help you prepare effectively for your A Level maths exams. The profit from every pack is reinvested into making free content on MME, which benefits millions of learners across the country.
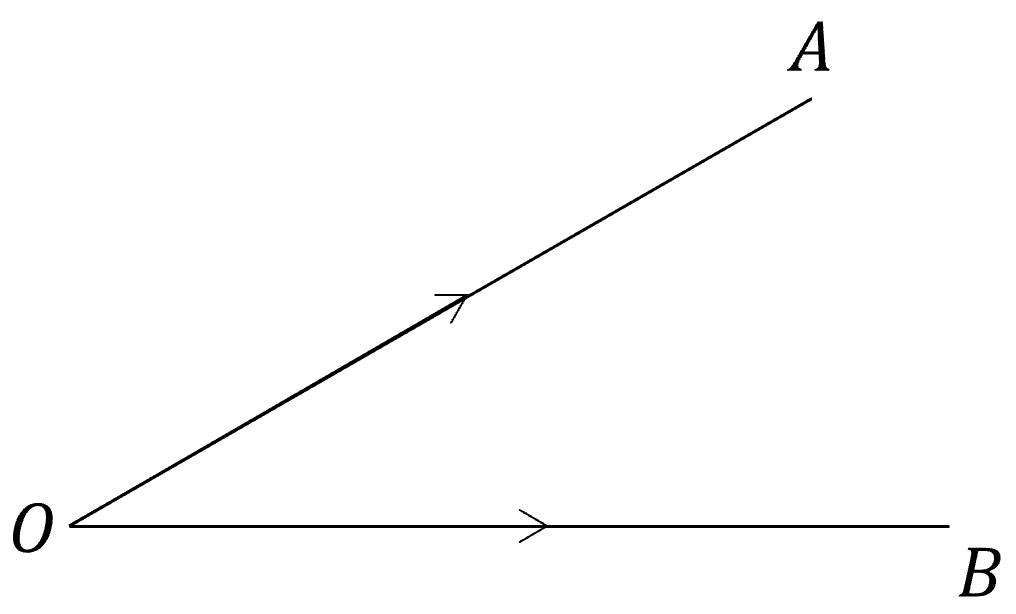
Working with Position Vectors
For a point A, the position vector is denoted as \overrightarrow{OA}
Likewise the point B is denoted as \overrightarrow{OB}
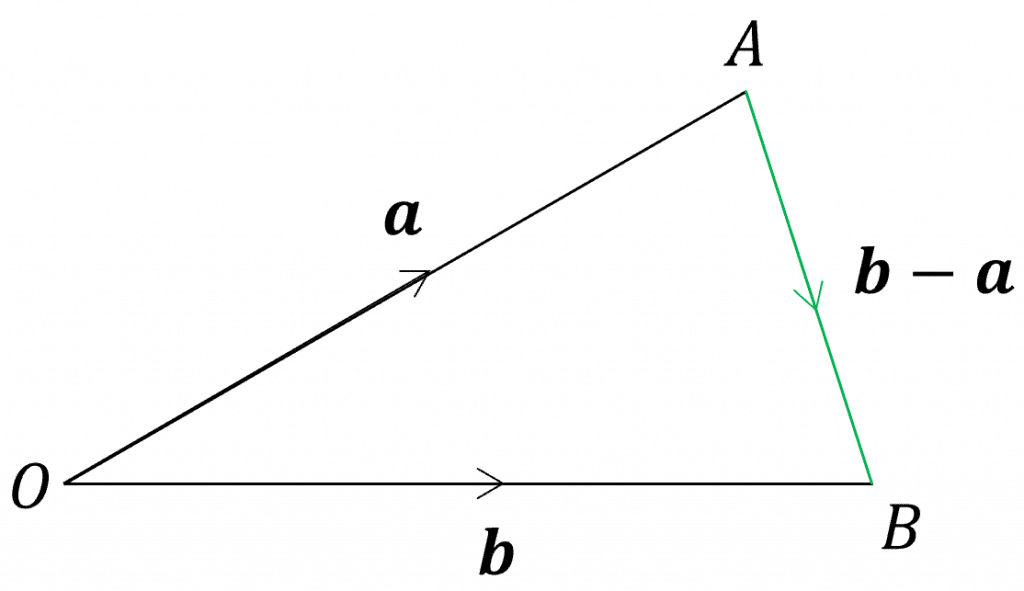
Writing points as position vectors then allows you to describe vectors between points as position vectors.
If we let, \boldsymbol{a}=\overrightarrow{OA} and \boldsymbol{b}=\overrightarrow{OB}
Then, \overrightarrow{AB}=-\overrightarrow{OA}+\overrightarrow{OB}=-\boldsymbol{a}+\boldsymbol{b}=\boldsymbol{b}-\boldsymbol{a}
Describing vectors using \boldsymbol{i} and \boldsymbol{j} Units
- A vector with a magnitude of 1 unit is called a unit vector
- The standard unit vectors are \boldsymbol{i} and \boldsymbol{j}, which are in the direction of the x-axis and y-axis respectively.
- These standard unit vectors are used to express the horizontal and vertical position of the end of a vector compared to its start point
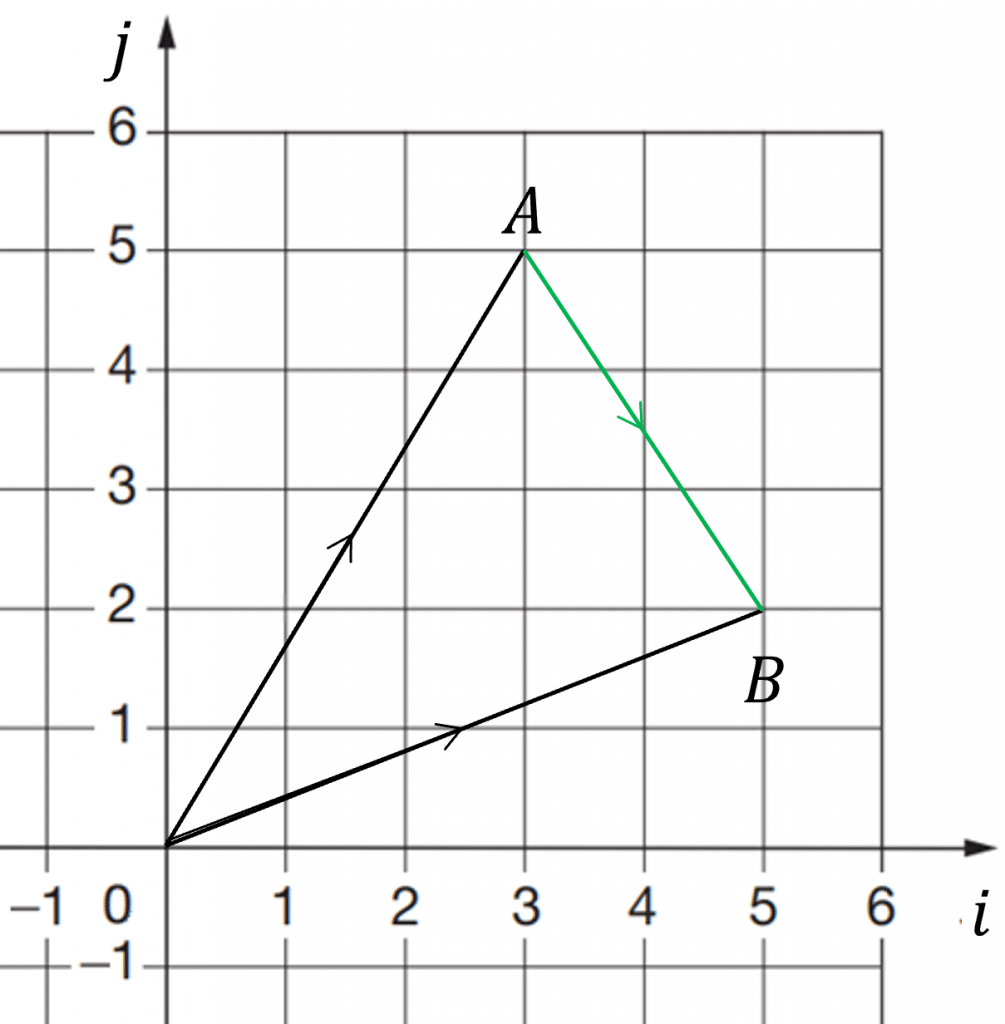
Using the diagram on the right, we can see the position vector of point A=3\boldsymbol{i}+5\boldsymbol{j} and the position vector of point B=5\boldsymbol{i}+2\boldsymbol{j}
To write \overrightarrow{AB} in terms of standard unit vectors, we can just add and subtract the \boldsymbol{i} and \boldsymbol{j} components of \boldsymbol{a} and \boldsymbol{b} separately.
Thus the vector \overrightarrow{AB}=-\boldsymbol{a}+\boldsymbol{b}=-(3\boldsymbol{i}+5\boldsymbol{j})+(5\boldsymbol{i}+2\boldsymbol{j})=2\boldsymbol{i}-3\boldsymbol{j}
This means that to go from A to B you go 2 units to the right and 3 units down.
Column Vectors
Column vectors are another way of expressing vectors.
If A=4\boldsymbol{i}-3\boldsymbol{j}, then the column vector for A is \dbinom{4}{-3}
To add and subtract column vectors from one another you simply add or subtract the top row and then add or subtract the bottom row. For example, if A=\dbinom{5}{-2} and B=\dbinom{1}{3}, then A+B=\dbinom{5+1}{-2+3}=\dbinom{6}{1}
Multiplying a column vector by a scalar is also straight forward, you just need to multiply both the top and bottom numbers by the scalar. For instance, if C=\dbinom{-3}{4}, 3C=\dbinom{3\times-3}{3\times4}=\dbinom{-9}{12}
Position Vectors Example Questions
Question 1: Point K has the coordinates (-1,3).
Give the position vector of point K in:
a) Standard unit vector form
b) Column vector form
[2 marks]
If A=(x,y), then A=x\boldsymbol{i}+y\boldsymbol{j}=\dbinom{x}{y}
So,
a) K=-\boldsymbol{i}+3\boldsymbol{j}
b) K=\dbinom{-1}{3}
Question 2: \boldsymbol{a} has a position vector of \dbinom{7}{-2} and \boldsymbol{b} has a position vector of \dbinom{-3}{-1}, what is 2\boldsymbol{a}-4\boldsymbol{b}?
Give your answer as a column vector.
[2 marks]
Question 3: Points X and Y have position vectors 5\boldsymbol{i}-\boldsymbol{j} and -3\boldsymbol{i}+4\boldsymbol{j} respectively. Point Z lies on the line XY, such that XZ:ZY=2:3.
Calculate the position vector of Z, giving your answer in standard unit form.
[4 marks]
We know that point Z lies on the line XY, such that XZ:ZY=2:3, so \overrightarrow{XZ}=\dfrac{2}{5}\overrightarrow{XY}
Therefore we can calculate the position of point Z from X:
\overrightarrow{XZ}=\dfrac{2}{5}(-8\boldsymbol{i}+5\boldsymbol{j})=-\dfrac{16}{5}\boldsymbol{i}+2\boldsymbol{j}Finally, add the position vector of \overrightarrow{XZ} to the position vector of X to get the position vector of Z (from the origin):
Z = 5\boldsymbol{i}-\boldsymbol{j}-\dfrac{16}{5}\boldsymbol{i}+2\boldsymbol{j}=\dfrac{9}{5}\boldsymbol{i}+\boldsymbol{j}You May Also Like...
MME Learning Portal
Online exams, practice questions and revision videos for every GCSE level 9-1 topic! No fees, no trial period, just totally free access to the UK’s best GCSE maths revision platform.
