Stationary Points

Finding Stationary Points

To find a point where the gradient is 0, we need to set \dfrac{dy}{dx} = f'(x) = 0.

So, let’s say we’ve got a nice, easy function: \textcolor{blue}{y = x^2 - 1}.

Well, we know that the gradient is given by \dfrac{dy}{dx} = 2x.

Setting \textcolor{limegreen}{\dfrac{dy}{dx} = 2x = 0} gives x = 0, for which y = 0^2 - 1 = -1.

We conclude that \textcolor{blue}{y = x^2 - 1} has a stationary point at (0, -1).

Finding Stationary Points of Complicated Functions

Let \textcolor{blue}{y = \sin x + \cos x} for x measured in radians. How might we go about finding the stationary points (in the range
0 \leq x \leq 2\pi)?

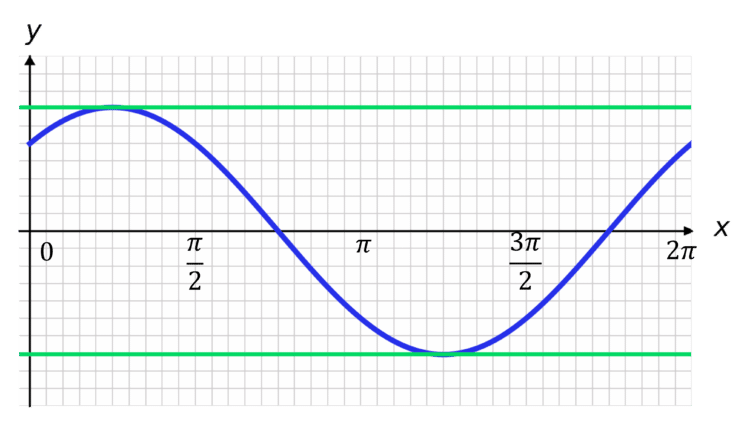
\textcolor{blue}{y = \sin x + \cos x} gives \dfrac{dy}{dx} = \cos x - \sin x.

Setting \textcolor{limegreen}{\dfrac{dy}{dx} = 0}, we require \cos x = \sin x, or, \tan x = 1.

This gives x = \tan ^{-1}(1) = \dfrac{\pi}{4}, \dfrac{5\pi}{4}.

Therefore, for 0 \leq x \leq 2\pi, we have the stationary points \left( \dfrac{\pi}{4}, \sqrt{2}\right) , \left( \dfrac{5\pi}{4}, -\sqrt{2}\right).

Finding the Nature of Stationary Points

Once we’ve found our stationary points, we need to find out whether they are a maximum, minimum, or a stationary point of inflection.

We do this by differentiating our derivative again.

So, for example, take our first example of y = x^2 - 1.

We said that \dfrac{dy}{dx} = 2x.

So \dfrac{d^{2}y}{dx^2} = 2.

Since \dfrac{d^{2}y}{dx^2} = 2 > 0, we can conclude that this is a minimum point. You probably figured that out from the graph, though.

What about the second example, y = \sin x + \cos x?

Well, we know that \dfrac{dy}{dx} = \cos x - \sin x.

By extension, \dfrac{d^{2}y}{dx^2} = -\sin x - \cos x. We now need to test both of our values of x for the corresponding value of \dfrac{d^{2}y}{dx^2}.

So, for x = \dfrac{\pi}{4}, \dfrac{d^{2}y}{dx^2} = -\sqrt{2} < 0, so it is a maximum point.

For x = \dfrac{5\pi}{4}, \dfrac{d^{2}y}{dx^2} = \sqrt{2} > 0, so it is a minimum point.

Sketching Graphs from Information about Functions

Say we have a complex function with multiple terms, i.e. \textcolor{blue}{f(x) = 1 + x - 2\cos x} where -2\pi \leq x \leq 2\pi is measured in radians.

Then f'(x) = 1 + 2\sin x and f''(x) = 2\cos x.

Therefore, any stationary points will occur when \sin x = \dfrac{-1}{2}, so x = \dfrac{-5\pi}{6}, \dfrac{-\pi}{6}, \dfrac{7\pi}{6}, \dfrac{11\pi}{6}.

For x = \dfrac{-5\pi}{6}, f(x) = 0.114 and f''(x) = -\sqrt{3}, which is a maximum.

For x = \dfrac{-\pi}{6}, f(x) = -1.256 and f''(x) = \sqrt{3}, which is a minimum.

For x = \dfrac{7\pi}{6}, f(x) = 6.397 and f''(x) = -\sqrt{3}, which is a maximum.

For x = \dfrac{11\pi}{6}, f(x) = 5.028 and f''(x) = \sqrt{3}, which is a minimum.

We can sketch these parts onto a graph to give us a rough form.

We can be helped further in sketching by considering the behaviour of the graph as x gets really big or really small. We know that the graph roughly follows y=x+1, so as x gets really big so does y, and as x gets really small so does y. With this, we can finish our graph.