The Normal Distribution
The Normal Distribution Revision
The Normal Distribution
The normal distribution is a bell shaped curve that is symmetric about the mean. It is defined by its mean \mu and its standard deviation \sigma. If X is normally distributed, we write X\sim N(\mu,\sigma^{2}).
Facts About the Normal Distribution
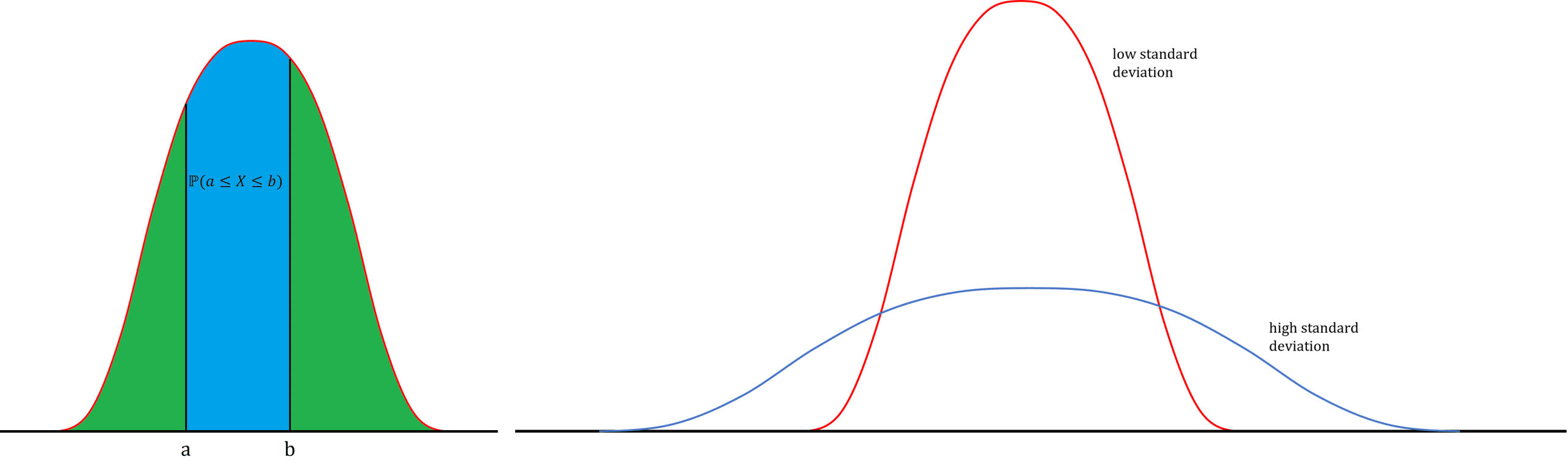
Probability is represented by the area under the graph (note this means the total area under the graph is 1).
The normal distribution is symmetric about the mean \mu, which means:
\mathbb{P}(X\geq\mu)=\mathbb{P}(X\leq\mu)=0.5
\mathbb{P}(X\geq\mu+a)=\mathbb{P}(X\leq\mu-a)\text{ for all }a
The standard deviation determines how flat the graph is – the higher the standard deviation, the lower the graph.
The graph tends to 0 as it travels away from the mean, but it never gets there.
Using a Calculator for the Normal Distribution
Your calculator might have a built in function for normal distribution probabilities.
This will ask you to put in a mean and standard deviation and an upper and lower bound on X to produce a probability.
This is fairly straightforward for questions such as \mathbb{P}(3\leq X\leq 6), but what about questions such as \mathbb{P}(X\leq 5), where there is no lower bound?
In this case, take the lower bound to be as low as your calculator allows you to input, so that it has as little effect on the result as possible.
Usually, -9999 for a lower bound or 9999 for an upper bound will suffice.
The Inverse Normal Function
Sometimes, you might be given a probability p and asked to find x such that p=\mathbb{P}(X<x).
For this, we use the inverse normal function, which should also be on your calculator.
You will input a mean, standard deviation and probability and the calculator gives you x such that p=\mathbb{P}(X<x).
Note: For < and \leq you can do this directly on your calculator. However, for > and \geq you need to subtract p from 1 to turn P(X > a) into P(X \leq a) = 1-p, then you can use your calculator as normal.
Example: The Normal Distribution
In the 2012 Olympics Men’s 100 metre sprint final, the average time taken was 10 seconds. The times were normally distributed with a variance of 0.2 seconds. Calculate the probability of a runner having finished the race in 9.58 seconds or less.
[2 marks]
X\sim N(10,0.2)Use your calculator, upper bound 9.58 and lower bound -9999
\mathbb{P}(X\leq 9.58)=0.1738The probability of a runner finishing the race in 9.58 seconds or less is 0.1738
The Normal Distribution Example Questions
Question 1: For X\sim N(4,1), calculate:
a) \mathbb{P}(X\leq 3.5)
b) \mathbb{P}(X\geq 6)
c) \mathbb{P}(X\leq 4.25)
[3 marks]
a) 0.3085
b) 0.0228
c) 0.5987
Question 2: For X\sim N(75,100), calculate:
a) \mathbb{P}(90\leq X\leq 110)
b) \mathbb{P}(65\leq X\leq 75)
c) \mathbb{P}(50\leq X\leq 100)
[3 marks]
a) 0.0666
b) 0.3413
c) 0.9876
Question 3: For X\sim N(20,25), find the value of a such that:
a) \mathbb{P}(X>a)=0.1
b) \mathbb{P}(X\leq a)=0.6
c) \mathbb{P}(15\leq X\leq a)=0.4
[4 marks]
a) a=26.41
b) a=21.27
c) a=20.74
Question 4: The weights of punnets of strawberries sold by a greengrocers are 250\text{ g} on average with a standard deviation of 9\text{ g}. What is the probability that Jenny gets a 280\text{ g} punnet or better from the grocers?
[2 marks]
We model this problem with X\sim N(250,81).
We want to find \mathbb{P}(X\geq 280)
\begin{aligned}\mathbb{P}(X\geq 280)&=1-\mathbb{P}(X\leq 280)\\[1.2em]&=1-\mathbb{P}(X\leq 280)\\[1.2em]&=1-0.9996\\[1.2em]&=0.0004\end{aligned}
Additional Resources
Exam Tips Cheat Sheet
A LevelFormula Booklet
A LevelYou May Also Like...

MME Learning Portal
Online exams, practice questions and revision videos for every GCSE level 9-1 topic! No fees, no trial period, just totally free access to the UK’s best GCSE maths revision platform.







